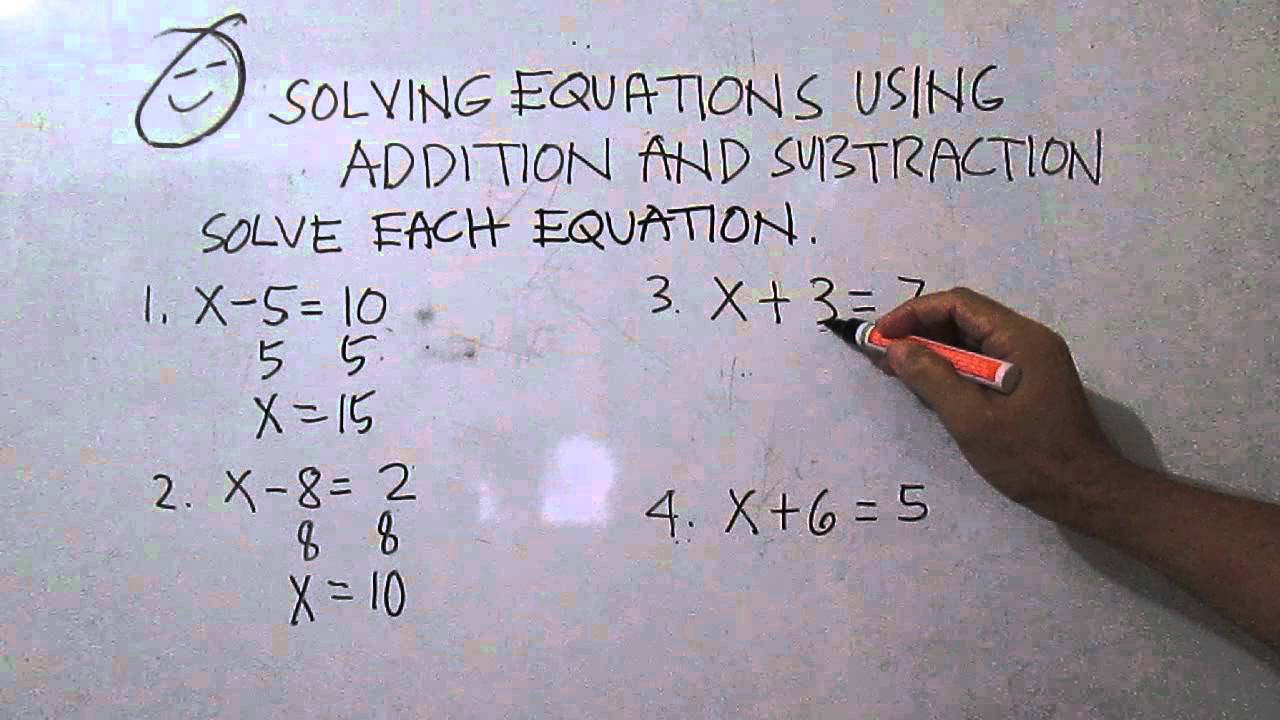Equation Wrangling: Mastering the Art of Adding and Subtracting Equations
Ever feel like you're juggling mathematical objects in the dark? Equations can seem like slippery things, especially when you need to combine them. But fear not, fellow equation wranglers! This guide will illuminate the path to mastering the art of adding and subtracting equations, a fundamental skill in the world of algebra and beyond.
Adding and subtracting equations is more than just a classroom exercise; it's a key to unlocking solutions in various fields, from physics and engineering to economics and computer science. Imagine balancing chemical reactions, calculating forces, or predicting market trends – equation manipulation lies at the heart of these endeavors.
The roots of manipulating equations can be traced back to ancient civilizations. Early mathematicians, like the Babylonians and Egyptians, developed methods for solving linear equations, laying the groundwork for the techniques we use today. These methods evolved through the contributions of Islamic scholars like Al-Khwarizmi and eventually reached Europe during the Renaissance. Over centuries, mathematicians refined these techniques into the modern algebraic methods we use today.
One of the main challenges in adding or subtracting equations is maintaining balance. Just like a seesaw, both sides of the equation must remain equal. Any operation performed on one side must also be performed on the other. This principle of equality is the bedrock of equation manipulation.
The fundamental concept is straightforward: to add or subtract equations, you perform the operation on the corresponding sides. For example, if you have two equations: x + 2y = 5 and 3x - y = 2, to add them, you combine like terms on each side: (x + 3x) + (2y - y) = 5 + 2, which simplifies to 4x + y = 7.
One benefit of equation combination is the elimination of variables. By strategically adding or subtracting equations, you can eliminate one variable, simplifying the problem and making it easier to solve for the remaining unknowns. This technique is invaluable in systems of equations.
Another benefit is the ability to find relationships between different equations. Combining equations can reveal hidden connections between variables, providing deeper insights into the system being studied. This can be particularly useful in scientific modeling and data analysis.
Finally, mastering equation manipulation enhances problem-solving skills. By learning to strategically manipulate equations, you develop critical thinking and analytical abilities applicable to a wide range of challenges, both in academics and everyday life.
An action plan for mastering these skills might involve starting with simple examples, gradually increasing complexity, and practicing regularly. Online resources, textbooks, and educational apps can provide a wealth of practice problems and step-by-step guidance.
Tips and Tricks
Always double-check your work to ensure both sides of the equation remain balanced.
If you are unsure, substitute your solution back into the original equations to verify its correctness.
Practice with diverse problems to solidify your understanding.
Advantages and Disadvantages of Manipulating Equations
| Advantages | Disadvantages |
|---|---|
| Solves systems of equations | Can be complex with multiple variables |
| Simplifies complex problems | Requires careful attention to detail to avoid errors |
FAQs
Q: Why is it important to maintain balance when adding or subtracting equations?
A: Maintaining balance ensures the equality of the equation is preserved, leading to a valid solution.
Q: Can I multiply or divide equations?
A: Yes, as long as you perform the same operation on both sides.
Q: How do I know which variable to eliminate?
A: Choose the variable whose coefficients are easiest to manipulate to cancel each other out.
Q: What are some common mistakes to avoid?
A: Forgetting to perform the operation on both sides, incorrectly combining like terms, and sign errors are common pitfalls.
Q: Where can I find more practice problems?
A: Textbooks, online resources, and educational apps offer ample practice materials.
Q: How is this applicable in the real world?
A: This is used in physics, engineering, economics, and various other fields.
Q: What if I get stuck?
A: Consult a teacher, tutor, or online resources for assistance.
Q: Are there alternative methods for solving systems of equations?
A: Yes, methods like substitution and graphing are also commonly used.
In conclusion, adding and subtracting equations is a powerful tool in the mathematician's arsenal. By understanding the fundamental principles, practicing diligently, and utilizing available resources, you can conquer the challenges of equation manipulation. This skill opens doors to solving complex problems, uncovering hidden relationships, and strengthening your overall analytical abilities. From scientific breakthroughs to everyday problem-solving, the ability to manipulate equations empowers you to explore the intricate world of mathematics and its applications. So, embrace the challenge, practice regularly, and unlock the potential of algebraic thinking! By mastering this skill, you'll not just be solving equations, you'll be unlocking a world of possibilities.
Unleash your inner fire exploring the world of llamas de fuego dibujo
The allure of vestido de la reina a journey through fashion and culture
Transform your bathroom with tub sliding glass shower doors
