Unlocking Circular Motion: Your Guide to AP Physics 1
Have you ever wondered what keeps a roller coaster car glued to the track as it loops upside down? Or how planets stay in orbit around the sun? The answer lies in the fascinating world of circular motion, a cornerstone concept in AP Physics 1. This guide will walk you through the essential formulas, concepts, and problem-solving strategies you need to conquer this topic.
Circular motion describes the movement of an object along a circular path. In AP Physics 1, we primarily focus on uniform circular motion, where the object moves at a constant speed. While the speed is constant, the velocity is not, because velocity is a vector quantity that includes direction. Since the direction is constantly changing in circular motion, so is the velocity. This change in velocity results in acceleration, specifically centripetal acceleration, which always points towards the center of the circle.
The core of understanding uniform circular motion lies in grasping centripetal force and acceleration. Centripetal force is the net force that causes an object to move in a circle. It isn't a new or separate type of force; rather, it's the resultant force acting towards the center. This force can be gravity, tension in a string, friction, or a combination of forces. Without a centripetal force, an object would move in a straight line, as dictated by Newton's First Law of Motion.
The historical understanding of circular motion has its roots in ancient astronomy. Observing the movement of celestial bodies sparked curiosity about the nature of these orbits. Early astronomers, despite lacking the tools we have today, made remarkable progress in understanding circular patterns in the sky. Over time, scientists like Newton formalized these observations into mathematical relationships, allowing us to predict and understand circular motion quantitatively.
Mastering circular motion formulas is essential for success in AP Physics 1. It's a foundational topic that connects to other areas like energy conservation and rotational motion. Common challenges students face include distinguishing between speed and velocity, understanding the direction of centripetal acceleration, and identifying the forces responsible for circular motion. This guide addresses these challenges and provides practical tips for overcoming them.
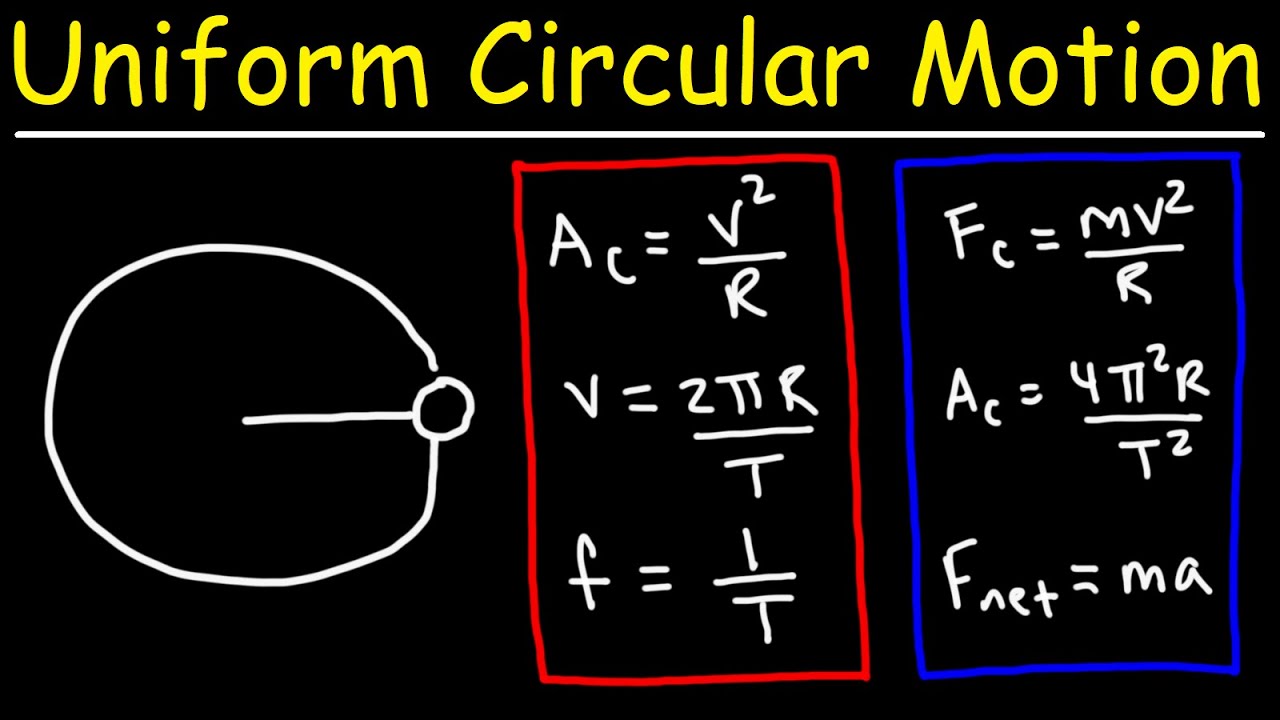
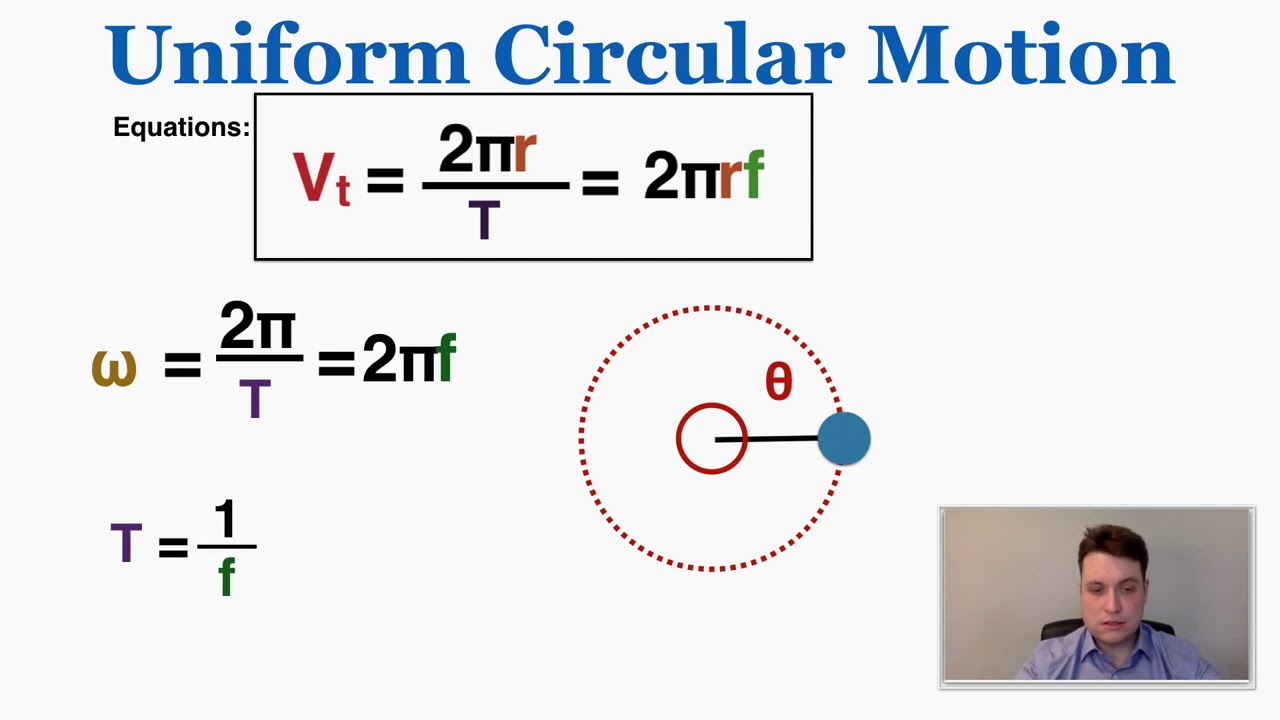
Key formulas for uniform circular motion include: * ac = v2/r (centripetal acceleration) * Fc = mac = mv2/r (centripetal force)
* v = 2πr/T (speed where T is the period, or time for one revolution)
These equations relate centripetal acceleration (ac), centripetal force (Fc), speed (v), radius (r), and the period of rotation (T). For example, if you know the speed and radius of a car moving in a circular path, you can calculate its centripetal acceleration. Similarly, if you know the mass and centripetal acceleration, you can find the centripetal force.Imagine a ball attached to a string, swung in a horizontal circle. The tension in the string provides the centripetal force that pulls the ball inwards, preventing it from flying off in a straight line. If you increase the speed of the ball, the tension in the string also increases to provide the greater centripetal force needed to maintain the circular path. This basic example demonstrates how the concepts and formulas work together.
Benefits of understanding these formulas include being able to analyze a wide range of real-world scenarios: the motion of cars on curved roads, the design of roller coasters, and the orbits of satellites. These concepts even extend to subatomic particles moving in circular accelerators.
An action plan for mastering circular motion involves practicing problem-solving. Start with simple examples and gradually work your way up to more complex scenarios. Identify the given quantities, determine what you're trying to find, and choose the appropriate formulas. Pay attention to units and draw diagrams to visualize the problem. Resources such as your textbook, online simulations, and practice problems are invaluable.
Advantages and Disadvantages of Focusing Solely on Uniform Circular Motion in an Introductory Course
| Advantages | Disadvantages |
|---|---|
| Provides a foundational understanding of circular motion principles | Doesn't cover non-uniform circular motion where speed changes. |
| Relatively simpler mathematically than non-uniform cases | Oversimplifies some real-world scenarios which are rarely perfectly uniform. |
Frequently Asked Questions
What is the difference between speed and velocity in circular motion?
Why does centripetal acceleration point towards the center?
What are some real-world examples of centripetal force?
How can I calculate the period of an object in circular motion?
What happens if the centripetal force is removed?
How does the radius affect centripetal acceleration?
What is the relationship between centripetal force and gravitational force in satellite motion?
How does friction contribute to the centripetal force in a car turning a corner?
In conclusion, understanding circular motion is a crucial step in your AP Physics 1 journey. From amusement park rides to the motion of planets, this concept explains a vast array of phenomena. By mastering the key formulas, understanding the underlying principles, and practicing problem-solving, you can unlock a deeper understanding of the physical world around us. This knowledge will not only help you excel in your AP Physics 1 course, but also equip you with the tools to appreciate and analyze the intricate dance of objects in circular motion. Begin practicing with problems today and witness the power of these concepts unfold.
Conquering week 7 decoding fantasy football player rankings
Shaggy chic the ultimate guide to medium shaggy hairstyles for women over 60
Unlock your potential the elm tree of mastery
