Unlocking the Secrets of 40: Exploring its Factors and Multiples
Ever found yourself pondering the building blocks of numbers? Maybe you're dividing a cake into equal slices, arranging flowers in a bouquet, or simply curious about the hidden structure within mathematics. Today, we're going on a journey to explore the divisors of 40, uncovering their significance and practical applications.
What exactly are divisors, you might ask? They're the numbers that divide evenly into another number, leaving no remainder. Think of it like perfectly fitting puzzle pieces. In our case, we're searching for those numerical pieces that fit snugly into 40. So, what numbers divide 40 perfectly? Let's find out!
The factors of 40 – another term for divisors – are the whole numbers that multiply together to give us 40. Discovering these factors opens a window into the fundamental relationships between numbers. It's a bit like discovering the secret ingredients of a recipe – understanding the components allows us to manipulate and create something new.
Why should we care about the divisors of 40? Well, understanding factors and divisibility has surprising real-world connections. From dividing resources equally to optimizing designs, these concepts underpin many practical applications. For example, imagine planning a party for 40 guests. Knowing the factors of 40 helps determine how to arrange tables and seating efficiently.
The quest for the divisors of 40 is more than just a mathematical exercise. It's a glimpse into the elegant patterns that govern the numerical world. By breaking down 40 into its fundamental components, we gain a deeper appreciation for the interconnectedness of mathematics and its relevance in our everyday lives. This exploration also lays the groundwork for understanding more complex mathematical concepts like prime factorization and least common multiples.
Finding the divisors of 40 involves identifying all the numbers that divide 40 without leaving a remainder. These are 1, 2, 4, 5, 8, 10, 20, and 40. This list represents the complete set of factors of 40.
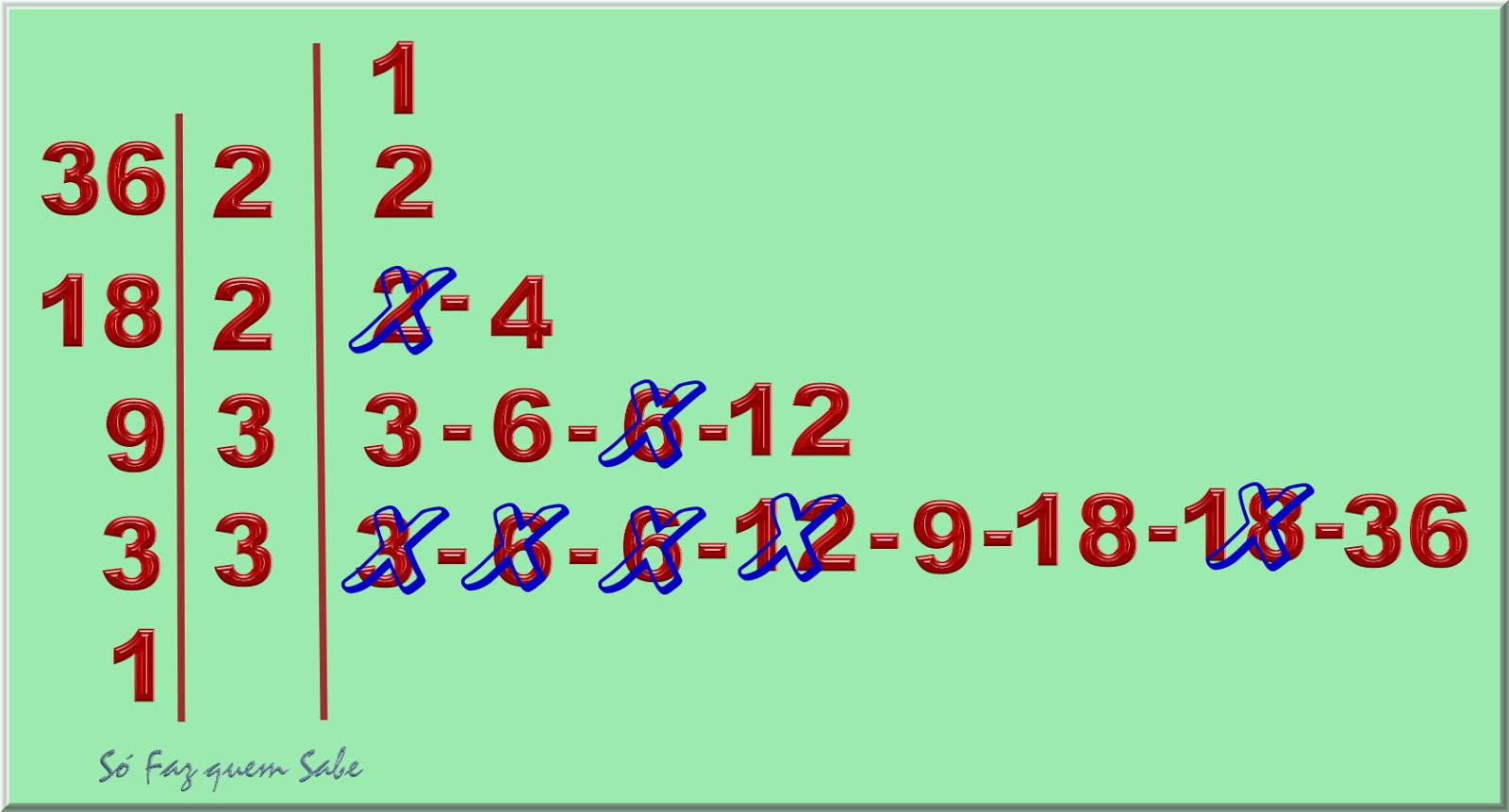
Prime factorization involves breaking down a number into its prime factors. For 40, the prime factorization is 2 x 2 x 2 x 5, or 2³ x 5. This representation highlights the essential prime numbers that make up 40.
One benefit of understanding divisors is efficient resource allocation. If you have 40 items to distribute equally, knowing the factors helps determine the possible group sizes.
Another benefit lies in simplifying fractions. Recognizing the common factors between the numerator and denominator allows for simplification. For example, the fraction 20/40 can be simplified to 1/2 by dividing both by the common factor 20.
Divisors also play a role in understanding patterns and relationships between numbers. This knowledge is fundamental in fields like cryptography and computer science.
To find the factors of a number, start by dividing by 1 and continue checking divisibility by progressively larger integers. Any number that divides evenly is a factor.
Advantages and Disadvantages of Working with Divisors
| Advantages | Disadvantages |
|---|---|
| Facilitates resource allocation | Can be time-consuming for large numbers |
| Simplifies fractions | No inherent disadvantages, but understanding the concept is crucial |
| Fundamental to understanding number theory |
Best Practices:
1. Start with 1 and the number itself: These are always factors.
2. Check divisibility by 2: If the number is even, 2 is a factor.
3. Continue checking divisibility by prime numbers: This is an efficient way to identify factors.
4. Use a factor tree: This visual representation can help break down the number into its prime factors.
5. Check for pairs of factors: Once you find a factor, its corresponding pair can be determined by dividing the original number by the factor.
Real Examples:
1. Dividing 40 candies equally among friends.
2. Simplifying the fraction 20/40.
3. Finding the dimensions of a rectangle with an area of 40.
4. Understanding the rhythmic patterns in music (e.g., a 40-beat sequence).
5. Designing a garden with 40 plants, arranged in equal rows.
FAQ:
1. What is a divisor? A number that divides another number evenly.
2. What are the factors of 40? 1, 2, 4, 5, 8, 10, 20, and 40.
3. What is prime factorization? Expressing a number as a product of prime numbers.
4. How can I find the divisors of a number? By systematically checking divisibility by integers.
5. Why are divisors important? They have applications in various fields, including resource allocation and simplifying fractions.
6. What is the relationship between factors and multiples? Factors divide a number evenly, while multiples are the products of a number and any integer.
7. Are all divisors of a number also factors? Yes, the terms "divisor" and "factor" are interchangeable.
8. Is 1 always a factor of any number? Yes, 1 is a factor of every number.
Tips and Tricks:
Use divisibility rules to quickly check if a number is a factor. For example, if a number ends in 0 or 5, it's divisible by 5.
In conclusion, exploring the divisors of 40 is a journey into the fundamental principles of number theory. From simplifying fractions to understanding the building blocks of numbers, these concepts have a wide range of applications. By recognizing the factors of 40 – 1, 2, 4, 5, 8, 10, 20, and 40 – we gain a deeper appreciation for the interconnectedness of mathematics and its practical significance. Whether you're dividing a cake, arranging objects, or simply curious about the numerical world, understanding divisors empowers you with a valuable tool for problem-solving and critical thinking. So, next time you encounter a number, take a moment to consider its divisors – you might be surprised by what you discover! Continue exploring, keep questioning, and let the world of numbers unfold before you. There's a universe of patterns waiting to be revealed.
The elusive quest for the perfect youtube name a journey through random username generators
Elevate your tiktok game how to seamlessly incorporate pictures
Drowning in a sea of creativity your guide to local art festivals this week
