Unlocking the Secrets of Triangles: Your Guide to Navigating Trigonometry Word Problems
Imagine standing at the foot of a majestic mountain, its peak shrouded in mist. You know the distance you need to traverse and the angle of ascent, but how do you determine the mountain's height? This, my friends, is where the magic of trigonometry comes into play.
Trigonometry, often perceived as a labyrinth of sines, cosines, and tangents, is essentially the study of relationships between angles and sides in triangles. While seemingly abstract, its principles underpin countless real-world phenomena, from architecture and engineering to music and astronomy. And what better way to bridge the gap between theoretical knowledge and practical application than through the art of solving trigonometry word problems?
Trigonometry word problems, unlike their equation-based counterparts, present challenges wrapped in narrative. They demand not just a mastery of formulas but also the ability to translate textual information into visual representations, namely triangles. This process of deciphering, strategizing, and ultimately arriving at a solution is where the true beauty of trigonometry lies.
But fear not, aspiring mathematicians, for within these problems lie invaluable opportunities for growth. Each solved problem serves as a stepping stone, bolstering your understanding of trigonometric concepts and sharpening your problem-solving prowess. Moreover, the sense of accomplishment derived from conquering a particularly intricate problem is a reward in itself.
This journey into the world of trigonometry word problems is not one you have to undertake alone. A plethora of resources awaits, from comprehensive textbooks and online tutorials to interactive worksheets and educational apps. These tools, coupled with a healthy dose of perseverance and a dash of curiosity, will equip you with the skills needed to not only solve these problems but to also appreciate the elegance and practicality of trigonometry in our everyday lives.
Let's delve deeper into the practical aspects of tackling these problems. One of the first steps involves identifying the 'givens' and the 'unknowns' within the word problem. What information has been provided, and what are you tasked with finding? Once you've deciphered this, sketch a triangle and label its sides and angles accordingly, ensuring your diagram accurately reflects the problem's context.
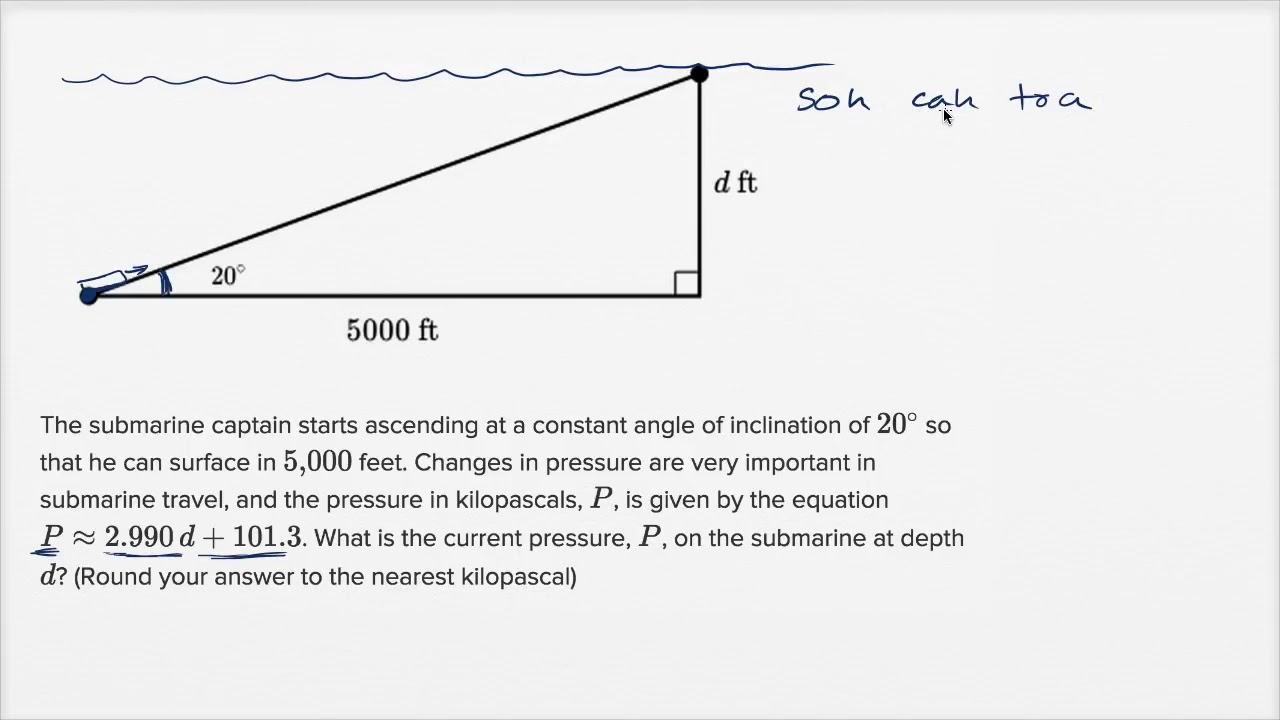
Next comes the crucial step of selecting the appropriate trigonometric ratio – sine, cosine, or tangent – to employ. This decision hinges on the specific information given and what needs to be determined. Remember SOH CAH TOA, your mnemonic friend, to guide your choice.
With the correct ratio chosen, set up an equation that links the given values to the unknown. Now, it's time to put your algebra skills to the test and solve for the unknown variable. Don't forget to consider units and round off your answer appropriately, paying attention to the level of precision required by the problem.
Remember, practice makes perfect. The more problems you tackle, the more confident you'll become in your ability to dissect, analyze, and ultimately conquer these trigonometric challenges. Embrace the journey, and soon you'll find yourself navigating the world of triangles with newfound ease and mastery.
Epic male dragon names a guide to choosing the perfect moniker
Acronym for legal writing
Navigating marriage meaningful conversations